Differentiation Formulas - Concept, Rules, Important Formula, Examples
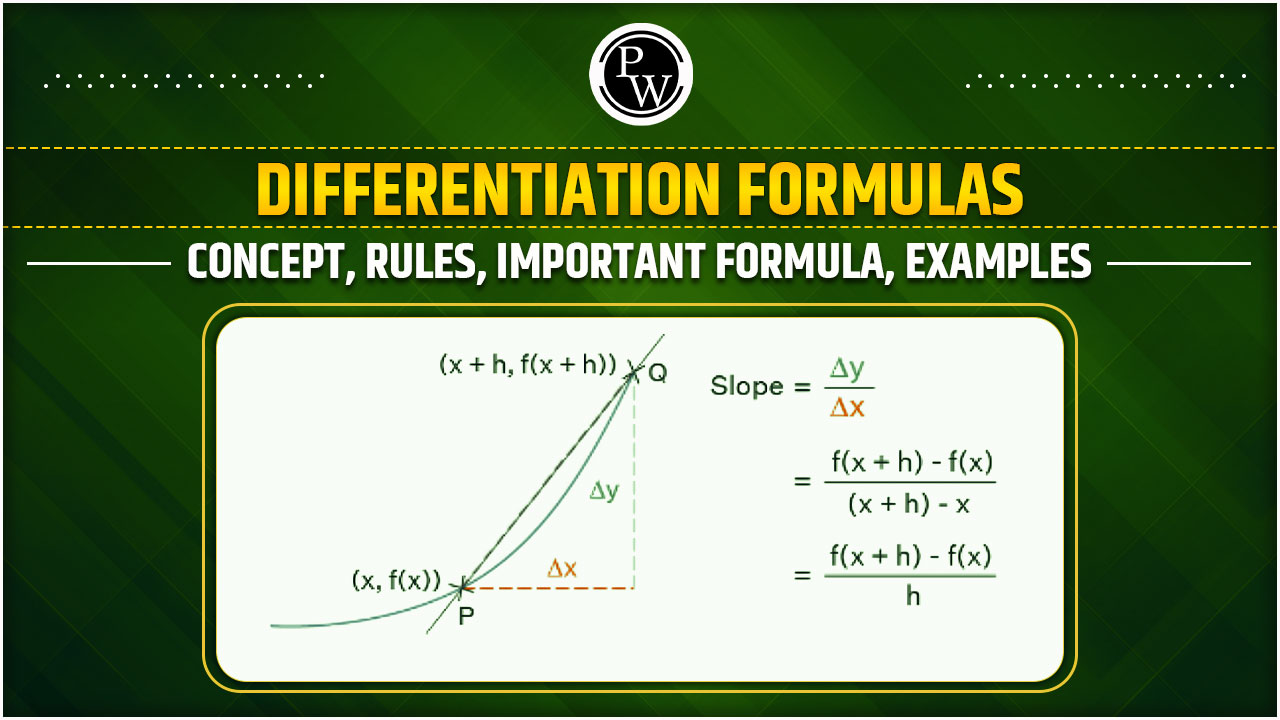
Differentiation Formulas:- A list of differentiation formulas is available for students to use as a reference when solving differential equations. Differentiation is a key topic in higher-level mathematics, and the derivative is commonly represented as d/dx.
This formula list covers derivatives for various types of functions, including constants, trigonometric functions, polynomials, hyperbolic functions, logarithmic functions, exponential functions, and inverse trigonometric functions. These formulas are essential for tackling numerous examples and problems found in the Class 11 and 12 curricula, helping students to solve them more effectively.
Check out: CBSE Class 12th Books
Differentiation Formulas
Here are some essential differentiation formulas covered in Class 11. For a function f(x)f(x)f(x), its derivative is denoted as f′(x)f'(x)f′(x):
Check Out: Class 12th Question Banks
Rules of Differentiation
The fundamental differentiation rules that are essential for solving various types of problems in calculus are outlined below:
Product Rule
Product Rule – When differentiating a function that is the product of two functions, say a(x)a(x)a(x) and b(x)b(x)b(x), the product rule is used.
This rule allows us to find the derivative of products of functions by taking the derivative of each function separately and applying a specific formula to combine them.
Sum and Difference Rule
Sum and Difference Rule – This rule applies when differentiating a function that is either a sum or difference of two functions. If f(x)f(x)f(x) is the sum of a(x)a(x)a(x) and b(x)b(x)b(x), then:
These rules indicate that the derivative of a sum is the sum of the derivatives, and the derivative of a difference is the difference of the derivatives.
Check Out: Class 12th Sample Papers
Chain Rule
Chain Rule – The chain rule is used for differentiating composite functions. If you have a function then according to the chain rule for differentiation,
This rule is crucial when dealing with nested functions or functions composed of other functions.
Quotient Rule
Quotient Rule – When differentiating a function that is the quotient of two functions, say a(x) divided by b(x), the quotient rule applies. If
This rule provides a way to find the derivative of a function that is the ratio of two other functions.
Combining Rules for Complex Functions
For functions that involve combinations of these basic types—sum, product, or quotient—the rules can be applied as follows:
Check out: School Books
Differentiation Formulas FAQs
1. What is the basic formula for differentiating a constant function?
Answer: The derivative of a constant function f(x), where k is a constant, is always 0.
f′(x)=0
2. How do you differentiate a power function?
Answer: For a power function f(x)=xn, where n is any real number, the derivative is given by:
3. What is the derivative of exe^xex?
Answer: The derivative of ex is ex is itself.










