Surface Areas and Volumes Class 10 Notes Maths Chapter 13

Home

Author at PW

March 10, 2025

Surface Areas and Volumes Class 10 Notes Maths Chapter 13: Students find surface areas and volumes challenging. Surface Areas and Volumes might seem challenging at first, but they are useful in everyday life! From calculating how much paint you need for your room to understanding the capacity of water tanks, these concepts are everywhere in your daily life.

Without a clear understanding, practical problems like calculating material usage or capacities remain confusing. We will simplify the topic with easy-to-follow explanations, essential formulas, and examples to help students confidently master Chapter 13 of Class 10 Maths.

Check out: CBSE Class 10th Books

Surface Area and Volume Class 10 Notes

Surface areas and volumes involve calculating the outer surface and the space occupied by 3D objects. These calculations are critical for solving practical problems, such as determining the amount of material required to construct an object or the capacity of a container. In this chapter, students learn the formulas and methods to calculate the surface area and volume of various geometric shapes, including cubes, cuboids, cylinders, cones, spheres, and hemispheres.

Check out: CBSE Class 10th Sample Papers

Class 10 surface area and volume formulas

Surface Area: The total area of the outer surfaces of a 3D object. For instance, the surface area of a cube is the sum of the areas of its six faces.
Volume: The amount of space occupied by a 3D object, often expressed in cubic units.
Applications: Understanding surface area and volume is essential in architecture, engineering, packaging, and daily life scenarios, such as calculating paint for walls or water in a tank.

Surface Area and Volume Class 10 Formulas

Memorizing the formulas for surface areas and volumes is crucial for solving problems quickly and accurately. Below are the key formulas for various shapes, cross-verified with reliable references to ensure accuracy and completeness:

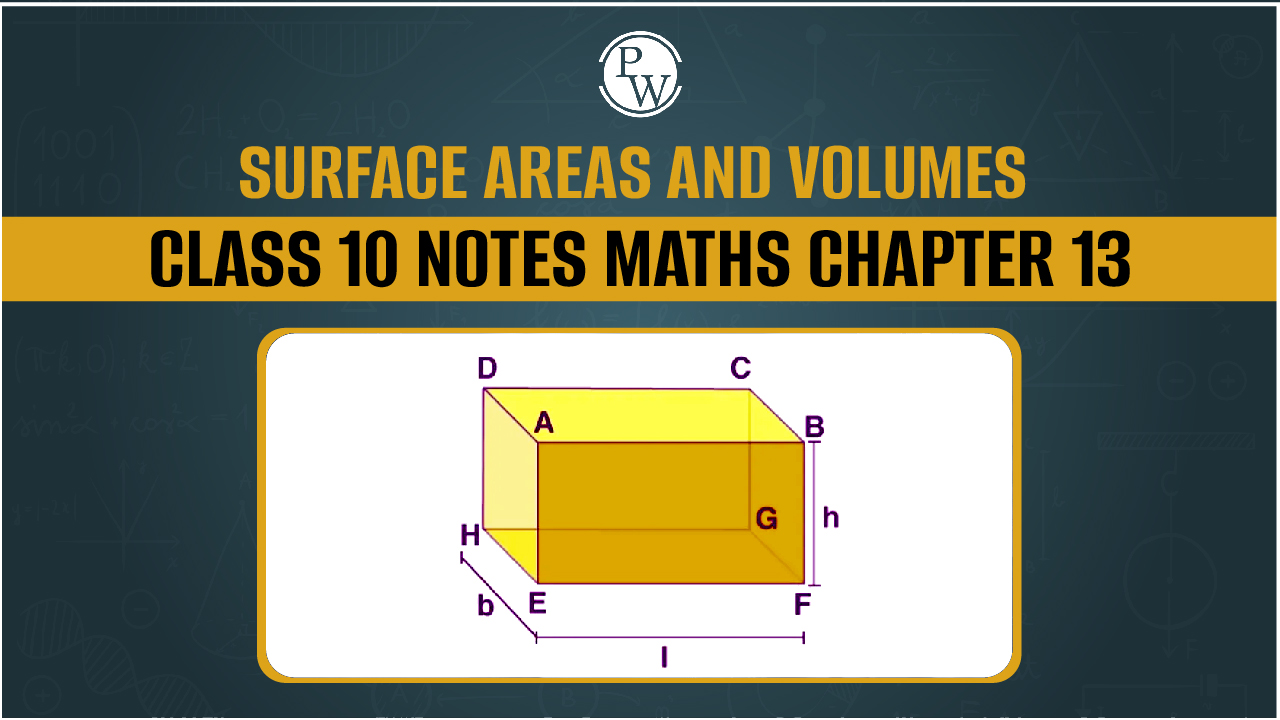
The total surface area of the cuboid (TSA) = Sum of the areas of all its six faces

TSA (cuboid) = 2(l × b) + 2(b × h) + 2(l × h) = 2(lb + bh + lh)

Lateral surface area (LSA) is the area of all the sides apart from the top and bottom faces.

The lateral surface area of the cuboid = Area of face AEHD + Area of face BFGC + Area of face ABFE + area of face DHGC

LSA (cuboid) = 2(b × h) + 2(l × h) = 2h(l + b)

Length of diagonal of a cuboid =√(l2 + b2 + h2)

Volume of a Cuboid

The area that is occupied by a cuboid’s six rectangular faces is its volume.

Volume of a cuboid = (base area) × height = (lb)h = lbh

Surface Area and Volume of Cube

Three-dimensional solids with six square faces, twelve edges, and eight vertices are called cubes.

Surface Area of Cube

As we know, one of the important properties of a cube is length = breadth = height.

If we assume that the length of the cube is “l”, and hence we get

l = breadth = height

So, here we get,

Breadth = l

Height = l

Cube with length l

The total surface area of the cube (TSA) = Sum of the areas of all its six faces.

In case of all faces has an equal area, TSA of Cube = 6 × area of Square = 6l2 square units.

Similarly, the Lateral surface area of cube = 2(l × l + l × l) = 4l2
Note: Diagonal of a cube =√3l

Check out: CBSE Class 10 Real Feel Sample Papers 2025

Formulas for Cones

Cone with Base Radius rand Height h:

Curved Surface Area (CSA):

CSA=πrl

Where l is the slant height.

Total Surface Area (TSA):
TSA=CSA+Area of Base=πrl+πr2=πr(l+r)

2. Volume:

1/3pi r^2 h

Sphere with Radius r:

Surface Area:
Surface Area=4πr2
Volume:
Volume=4/3πr3

These formulas collectively form the class 10 surface area and volume formulas, which are vital for mastering this chapter. For example, knowing the surface area formula of a cylinder (2πr(r + h)) can help calculate the material required to make a cylindrical container, while the volume formula (πr²h) is useful for determining the container's capacity to store liquids. These practical applications make understanding these formulas essential for solving real-world problems.

Volume of a Cube

Volume of a cube = base area × height
Since all dimensions of a cube are identical, volume = l3
Where l is the length of the edge of the cube.

Check out: CBSE Class 10th Question Banks

Surface Area and Volume of Cylinder

A cylinder is a solid form consisting of two circular bases joined by a lateral surface. A cylinder thus has three faces: one lateral, one round, and one circular. We can calculate a cylinder’s volume and surface area using these measurements.

Surface Area of the Cylinder

Consider a cylinder with height h and base radius r in units. When the curved surface of this cylinder is opened along the circular base’s diameter (d = 2r), it can be turned into a rectangle with dimensions of h units for height and 2πr for length. Consequently,

Transformation of a Cylinder into a rectangle.

CSA of a cylinder of base radius r and height h = 2π × r × h
TSA of a cylinder of base radius r and height h = 2π × r × h + area of two circular bases
=2π × r × h + 2πr2
=2πr(h + r)

Hemisphere with Radius r:

Curved Surface Area (CSA):

CSA=2πr2

Total Surface Area (TSA):

TSA=CSA+Area of Base Circle=2πr2+πr2=3πr2

Volume:

Volume=2/3πr3

Tricks to Memorize and Apply Formulas

Understand the Shapes: Visualize the 3D shapes to grasp the relationship between their dimensions and formulas.
Practice Regularly: Solve a variety of problems involving the surface area and volume class 10 notes to build confidence.
Create a Formula Chart: Keep a handy chart of the class 10 surface area and volume formulas for quick reference.
Apply in Real-Life Problems: Practice with real-world examples, such as calculating the paint needed for walls or the water capacity of a tank.

Common Mistakes to Avoid

Misinterpretation of Dimensions: Ensure you correctly identify the radius, height, or slant height.
Units Confusion: Always use consistent units and convert them when necessary.
Overlooking Curved vs Total Surface Area: Understand whether the problem requires CSA or TSA.

Check out: CBSE Class 10th Revision Books

Practice Questions

A cuboid has dimensions 5 cm, 4 cm, and 3 cm. Find its surface area and volume.
Calculate the volume of a cylinder with a radius of 7 cm and a height of 10 cm.
Find the TSA of a cone with a radius of 6 cm and a slant height of 10 cm.
A sphere has a radius of 14 cm. Find its surface area and volume.
A frustum of a cone has a radius of 5 cm and 3 cm and a height of 8 cm. Calculate its volume and curved surface area.
A hemispherical bowl has a radius of 10 cm. Determine its total surface area and volume.

These questions cover a range of problems designed to test your understanding of the surface area and volume class 10 notes and formulas.

Mastering the concepts of surface areas and volumes in Class 10 Maths Chapter 13 is critical for both academic and practical success. By focusing on consistent practice, creating a personalized formula chart, and applying the formulas to real-world scenarios such as calculating material usage or storage capacities, students can develop a deeper understanding This will enhance students' problem-solving skills. To excel in this topic, students should understand the derivations, practice the surface area and volume class 10 formulas, and apply them to solve real-world problems. Regular practice and clarity of concepts will ensure confidence during exams.

Check out: CBSE Class 10th Previous Year Papers