Understanding Heron’s Formula: Common Mistakes & Solutions

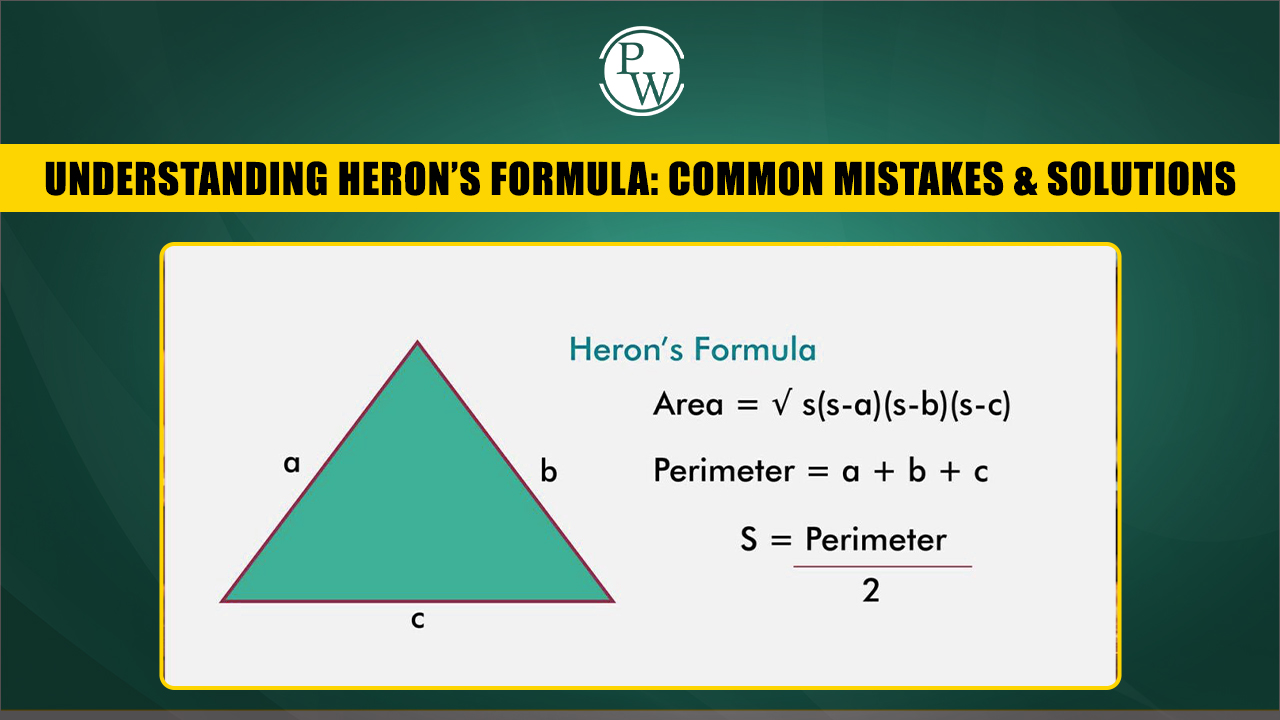
Heron's Formula:- Heron’s formula, also known as Heron’s triangle formula, is a valuable tool in geometry for finding the area of various types of triangles—whether they are equilateral, isosceles, or scalene—as well as certain quadrilaterals. So, what is Heron's formula? Simply put, it allows us to calculate the area of a triangle when the lengths of all its sides are known. This is achieved using the semi-perimeter of the triangle along with each side length. Heron's formula is a core part of the CBSE Class 9 curriculum, and there are even Heron’s formula calculators available to simplify the computation.

In this lesson, we will look into using Heron’s formula to determine the area of triangles and quadrilaterals. Through solved examples, you will gain a clear understanding of Heron’s formula and its practical application.

Check out: Class 9th Books

What is Heron’s Formula?

Heron’s formula, also known as Hero’s formula, is a useful method for calculating the area of a triangle when the lengths of all three sides are known. This approach is unique in that it does not rely on the triangle’s angles but instead solely on the side lengths. A key component in this formula is the term "s," or the semi-perimeter, which is calculated by taking half of the triangle’s perimeter. This concept also extends to finding the area of certain quadrilaterals by applying similar principles.

History of Heron's Formula

Heron’s formula, created around 60 CE by Heron of Alexandria, provides a way to calculate the area of a triangle using only the lengths of its sides. This approach, also referred to as Heron’s triangle formula, doesn’t require knowledge of the triangle’s angles. Heron, a Greek engineer and mathematician, further applied this method to find the area of quadrilaterals and used it to validate key trigonometric principles, such as the Law of Cosines and the Law of Cotangents. 

For students in Class 9, understanding Heron’s formula—or exploring tools like a Heron’s formula calculator—is essential for mastering area calculations. So, what is Heron’s formula exactly? It’s a technique that incorporates the semi-perimeter, "s," obtained by halving the triangle's perimeter, and this enables precise area measurement based solely on side lengths.

Also Check, Class 9 Sample Papers

Heron’s Formula for Triangles

Heron’s formula allows us to determine the area of any triangle—be it scalene, isosceles, or equilateral—as long as we know the lengths of its sides.

Consider a triangle ABC, with sides labelled a, b, and c. The area of this triangle can be calculated using Heron's formula:

where s is the semi-perimeter, defined as:

In this formula, a, b, and c represent the lengths of the triangle’s sides.

How to Find the Area Using Heron’s Formula?

To calculate the area of a triangle using Heron’s formula, follow these steps:

1. First, determine the perimeter of the triangle by adding the lengths of all three sides.

2. Next, calculate the semi-perimeter, s, using the formul:

3. Substitute s and the side lengths a, b, and c into Heron’s formula: 

4. Express the area in appropriate square units, such as

Heron's Formula for Equilateral Triangle

For an equilateral triangle, where all three sides are equal in length (let’s denote each side as a), Heron’s formula can be simplified. Since each side is the same, the formula becomes easier to apply:

1. Calculate the Semi-Perimeter:
   

2. Apply Heron’s Formula: Substitute s and aaa into the formula:
   
   For an equilateral triangle, this simplifies further to:
   

3. Final Simplified Area Formula: After simplifying, the area of an equilateral triangle using Heron’s formula is:
   

So, for any equilateral triangle, you can directly use this result:

Check out: Class 9th Revision Books

Heron's Formula for Scalene Triangle

In a scalene triangle, each side has a different length. Let the side lengths be a, b, and c, with the semi-perimeter represented by s and the area by A. The area A of a scalene triangle can be found using Heron’s formula:

where s is the semi-perimeter, calculated as:

This formula allows us to find the area using only the lengths of the triangle’s sides.

Heron's Formula for Isosceles Triangle

Heron’s formula can be applied to an isosceles triangle, where two sides are of equal length. Let the two equal sides be a, and the base be b. The semi-perimeter (s) and area (A) of the isosceles triangle can be calculated as follows:

Step 1: Calculate the Semi-Perimeter

The semi-perimeter s is given by:

Step 2: Apply Heron’s Formula

Using Heron’s formula, the area A of the triangle is:

Substitute the value of s into the formula:

Step 3: Simplify the Formula

After simplifying, we get the area of the isosceles triangle:

Conclusion

Thus, the area of an isosceles triangle using Heron’s formula is:

where a is the length of the two equal sides and b is the length of the base.

Check out: PW School Books

Applications of Heron's Formula

1. Finding the Area of Any Triangle

The most common application of Heron’s formula is to find the area of a triangle when the lengths of all three sides are known. This applies to all types of triangles, including:

• Scalene triangles (all sides of different lengths)

• Isosceles triangles (two equal sides)

• Equilateral triangles (all three sides equal)

Heron’s formula provides a way to calculate the area without needing to know the angles of the triangle.

2. Application in Geometry Problems

In geometry, many problems require the calculation of areas of triangles in various shapes. For instance, given the side lengths of a triangle inscribed in a circle or within a larger polygon, Heron's formula is often used to find the area directly.

3. Surveying and Land Measurement

Surveyors often use Heron’s formula to calculate the area of irregular plots of land. By measuring the lengths of the sides of a triangular parcel of land, the area can be quickly determined. This is particularly useful for land parcels that are not perfect rectangles or squares but can be approximated by triangles.

4. Involving Triangular Shapes in Engineering and Architecture

In engineering and architecture, when designing structures with triangular components, Heron’s formula can be used to compute areas for precise calculations in construction, like for trusses, roofs, and various components that rely on triangular geometry.

5. Triangular Meshes and Finite Element Analysis

In computational geometry and finite element analysis, triangular meshes are often used to model complex surfaces. Heron’s formula can be applied to compute the area of each triangle in the mesh, aiding in the calculation of surface areas and stresses in physical systems.

6. In Trigonometry Proofs

Heron’s formula is often used in trigonometric derivations and proofs. For example, it can be used to prove various trigonometric identities or calculate areas when working with certain types of triangles in trigonometry.

7. Finding the Area of a Quadrilateral

By dividing a quadrilateral into two triangles, Heron’s formula can also be used to find the area of irregular quadrilaterals, as long as the lengths of the sides are known. This method helps to simplify the complex problem of calculating the area of irregular shapes.

8. Application in Navigation and GPS

In navigation, Heron’s formula can be used to determine the area of a triangular region defined by three geographical points, which could be part of larger navigation and mapping systems.

9. Solving Real-World Problems in Physics

In physics, problems involving forces, pressures, or energies acting on triangular structures (like beams, bridges, and other engineering applications) may require the area of the triangle for further calculations, which Heron’s formula can provide.

10. Land Parcel Divisions

When dividing land into smaller triangular parcels, Heron’s formula helps to determine the area of each parcel based on the known lengths of the sides, assisting in equitable distribution and planning.

Check out: PW Stationery

Heron's Formula FAQs

1. What is Heron’s Formula?

Heron’s formula is a mathematical formula used to calculate the area of a triangle when the lengths of all three sides are known. It is named after the ancient Greek mathematician Heron of Alexandria.

2. Can Heron’s Formula be Used for All Types of Triangles?

Yes, Heron’s formula can be used to find the area of any type of triangle, whether it is scalene, isosceles, or equilateral, as long as the side lengths are known.

3. What if the Triangle is Right-Angled?

Heron’s formula can still be used for right-angled triangles, though you can also use the simpler formula 1/2×base×height to find the area directly.

4. How Accurate is Heron’s Formula?

Heron’s formula is highly accurate for calculating the area of a triangle as long as the side lengths are correctly measured. There are no inherent limitations in its accuracy.

5. Can Heron’s Formula Be Used for Quadrilaterals?

While Heron’s formula is specifically for triangles, it can be applied to quadrilaterals by dividing them into two triangles. Once you calculate the area of both triangles, you can add them together to find the total area of the quadrilateral.